Text Book of Conic Section
Free download. Book file PDF easily for everyone and every device. You can download and read online Text Book of Conic Section file PDF Book only if you are registered here. And also you can download or read online all Book PDF file that related with Text Book of Conic Section book. Happy reading Text Book of Conic Section Bookeveryone. Download file Free Book PDF Text Book of Conic Section at Complete PDF Library. This Book have some digital formats such us :paperbook, ebook, kindle, epub, fb2 and another formats. Here is The CompletePDF Book Library. It's free to register here to get Book file PDF Text Book of Conic Section Pocket Guide.
Book Reg. Product Description Product Details Illustrated with interesting examples from everyday life, this text shows how to create ellipses, parabolas, and hyperbolas and presents fascinating historical background on their ancient origins. The text starts with a discussion of techniques for generating the conic curves, showing how to create accurate depictions of large or small conic curves and describing their reflective properties, from light in telescopes to sound in microphones and amplifiers. It further defines the role of curves in the construction of auditoriums, antennas, lamps, and numerous other design applications.
Only a basic knowledge of plane geometry needed; suitable for undergraduate courses. Excursions in Geometry. Geometry: A Comprehensive Course. The Divine Proportion. Geometry from Euclid to Knots. The Beauty of Geometry: Twelve Essays. The most general equation is of the form [11]. The above equation can be written in matrix notation as [12]. This form is a specialization of the homogeneous form used in the more general setting of projective geometry see below.
If the conic is non-degenerate , then: [14]. In the notation used here, A and B are polynomial coefficients, in contrast to some sources that denote the semimajor and semiminor axes as A and B. Otherwise, assuming the equation represents either a non-degenerate hyperbola or ellipse, the eccentricity is given by. It can also be shown [17] : p. In the case of an ellipse the squares of the two semi-axes are given by the denominators in the canonical form. In polar coordinates , a conic section with one focus at the origin and, if any, the other at a negative value for an ellipse or a positive value for a hyperbola on the x -axis, is given by the equation.
Description:
The polar form of the equation of a conic is often used in dynamics ; for instance, determining the orbits of objects revolving about the Sun. Just as two distinct points determine a line, five points determine a conic. Formally, given any five points in the plane in general linear position , meaning no three collinear , there is a unique conic passing through them, which will be non-degenerate; this is true in both the Euclidean plane and its extension, the real projective plane.

Indeed, given any five points there is a conic passing through them, but if three of the points are collinear the conic will be degenerate reducible, because it contains a line , and may not be unique; see further discussion. Four points in the plane in general linear position determine a unique conic passing through the first three points and having the fourth point as its center.
Thus knowing the center is equivalent to knowing two points on the conic for the purpose of determining the curve. Any point in the plane is on either zero, one or two tangent lines of a conic. A point on just one tangent line is on the conic. A point on no tangent line is said to be an interior point or inner point of the conic, while a point on two tangent lines is an exterior point or outer point. All the conic sections share a reflection property that can be stated as: All mirrors in the shape of a non-degenerate conic section reflect light coming from or going toward one focus toward or away from the other focus.
In the case of the parabola, the second focus needs to be thought of as infinitely far away, so that the light rays going toward or coming from the second focus are parallel.
Account Options
Pascal's theorem concerns the collinearity of three points that are constructed from a set of six points on any non-degenerate conic. The theorem also holds for degenerate conics consisting of two lines, but in that case it is known as Pappus's theorem. Non-degenerate conic sections are always " smooth ". This is important for many applications, such as aerodynamics, where a smooth surface is required to ensure laminar flow and to prevent turbulence. It is believed that the first definition of a conic section was given by Menaechmus died BCE as part of his solution of the Delian problem Duplicating the cube.
Cones were constructed by rotating a right triangle about one of its legs so the hypotenuse generates the surface of the cone such a line is called a generatrix. Three types of cones were determined by their vertex angles measured by twice the angle formed by the hypotenuse and the leg being rotated about in the right triangle.
The conic section was then determined by intersecting one of these cones with a plane drawn perpendicular to a generatrix. The type of the conic is determined by the type of cone, that is, by the angle formed at the vertex of the cone: If the angle is acute then the conic is an ellipse; if the angle is right then the conic is a parabola; and if the angle is obtuse then the conic is a hyperbola but only one branch of the curve.
Euclid fl.
His main interest was in terms of measuring areas and volumes of figures related to the conics and part of this work survives in his book on the solids of revolution of conics, On Conoids and Spheroids. The greatest progress in the study of conics by the ancient Greeks is due to Apollonius of Perga died c. Apollonius's study of the properties of these curves made it possible to show that any plane cutting a fixed double cone two napped , regardless of its angle, will produce a conic according to the earlier definition, leading to the definition commonly used today.
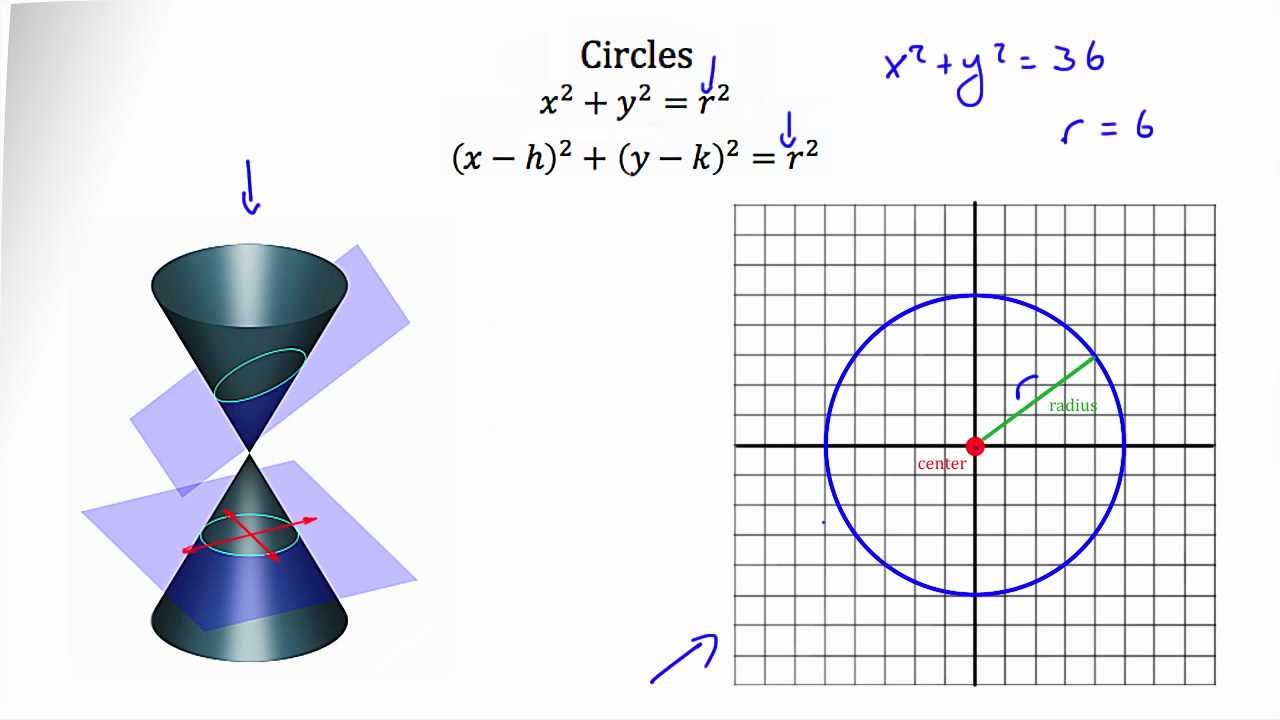
Circles, not constructible by the earlier method, are also obtainable in this way. This may account for why Apollonius considered circles a fourth type of conic section, a distinction that is no longer made. Apollonius used the names ellipse , parabola and hyperbola for these curves, borrowing the terminology from earlier Pythagorean work on areas.
Pappus of Alexandria died c. An instrument for drawing conic sections was first described in CE by the Islamic mathematician Al-Kuhi. Apollonius's work was translated into Arabic, and much of his work only survives through the Arabic version. Johannes Kepler extended the theory of conics through the " principle of continuity ", a precursor to the concept of limits.
Kepler first used the term foci in Girard Desargues and Blaise Pascal developed a theory of conics using an early form of projective geometry and this helped to provide impetus for the study of this new field.
- Childrens Language and Communication Difficulties: Understanding, Identification and Intervention (Continuum education).
- Level 3 Calculus - Conics - WikiEducator.
- From Secretion to Glaucom.
In particular, Pascal discovered a theorem known as the hexagrammum mysticum from which many other properties of conics can be deduced. This had the effect of reducing the geometrical problems of conics to problems in algebra. However, it was John Wallis in his treatise Tractatus de sectionibus conicis who first defined the conic sections as instances of equations of second degree. This work, which uses Fermat's methodology and Descartes' notation has been described as the first textbook on the subject.
Conic sections are important in astronomy : the orbits of two massive objects that interact according to Newton's law of universal gravitation are conic sections if their common center of mass is considered to be at rest.
Exchange Discount Summary If from the point of contact of a tangent to a parabola, a chord be drawn, and a line parallel to the axis meeting the chord, the tangent, and the curve, shew that this line will be divided by them in the same ratio as it divides the chord. Hence OQ. If the tangent at any point meets a pair of conjugate diameters in T and prove that T T 0 subtends supplementary angles at the foci. Our Blog. Hence the diameter through the middle point of a chord passes, when produced, through the point of intersection of the tangents at the ends of the chord. Math Formula Sheets.
If they are bound together, they will both trace out ellipses; if they are moving apart, they will both follow parabolas or hyperbolas. See two-body problem. The reflective properties of the conic sections are used in the design of searchlights, radio-telescopes and some optical telescopes.
The 4.
Text Book of Conic Section, Sharma.pdf
The conic sections have some very similar properties in the Euclidean plane and the reasons for this become clearer when the conics are viewed from the perspective of a larger geometry. The Euclidean plane may be embedded in the real projective plane and the conics may be considered as objects in this projective geometry. One way to do this is to introduce homogeneous coordinates and define a conic to be the set of points whose coordinates satisfy an irreducible quadratic equation in three variables or equivalently, the zeros of an irreducible quadratic form.
More technically, the set of points that are zeros of a quadratic form in any number of variables is called a quadric , and the irreducible quadrics in a two dimensional projective space that is, having three variables are traditionally called conics. The Euclidean plane R 2 is embedded in the real projective plane by adjoining a line at infinity and its corresponding points at infinity so that all the lines of a parallel class meet on this line.
On the other hand, starting with the real projective plane, a Euclidean plane is obtained by distinguishing some line as the line at infinity and removing it and all its points. In a projective space over any division ring, but in particular over either the real or complex numbers, all non-degenerate conics are equivalent, and thus in projective geometry one simply speaks of "a conic" without specifying a type.
That is, there is a projective transformation that will map any non-degenerate conic to any other non-degenerate conic. The three types of conic sections will reappear in the affine plane obtained by choosing a line of the projective space to be the line at infinity. The three types are then determined by how this line at infinity intersects the conic in the projective space.
In the corresponding affine space, one obtains an ellipse if the conic does not intersect the line at infinity, a parabola if the conic intersects the line at infinity in one double point corresponding to the axis, and a hyperbola if the conic intersects the line at infinity in two points corresponding to the asymptotes. In homogeneous coordinates a conic section can be represented as:. If the determinant of the matrix of the conic section is zero, the conic section is degenerate. As multiplying all six coefficients by the same non-zero scalar yields an equation with the same set of zeros, one can consider conics, represented by A , B , C , D , E , F as points in the five-dimensional projective space P 5.
Metrical concepts of Euclidean geometry concepts concerned with measuring lengths and angles can not be immediately extended to the real projective plane. This can be done for arbitrary projective planes , but to obtain the real projective plane as the extended Euclidean plane, some specific choices have to be made.